Why are so many ancient systems based 12? Because it is easy to do math with 12. According to Wikipedia:
12 is a highly composite number, the smallest number with four non-trivial factors (2, 3, 4, 6), and the smallest to include as factors all four numbers (1 to 4) within the subitizing range. As a result of this increased factorability of the radix and its divisibility by a wide range of the most elemental numbers (whereas ten has only two non-trivial factors: 2 and 5, with neither 3 nor 4), duodecimal representations fit more easily than decimal ones into many common patterns, as evidenced by the higher regularity observable in the duodecimal multiplication table. Of its factors, 2 and 3 are prime, which means the reciprocals of all 3-smooth numbers (such as 2, 3, 4, 6, 8, 9...) have a terminating representation in duodecimal. In particular, the five most elementary fractions (1⁄2, 1⁄3, 2⁄3, 1⁄4 and 3⁄4), all have a short terminating representation in duodecimal (0.6, 0.4, 0.8, 0.3 and 0.9, respectively), and twelve is the smallest radix with this feature (since it is the least common multiple of 3 and 4). This all makes it a more convenient number system for computing fractions than most other number systems in common use, such as the decimal, vigesimal, binary, octaland hexadecimal systems, although the sexagesimal system (where the reciprocals of all 5-smooth numbers terminate) does better in this respect (but at the cost of an unwieldily large multiplication table).
Here’s why so many ancient system are based 12 – they are simpler to compute. And this is why we still have 12 hours cycles. And this is probably why the non-scientific community took longer to give base 12 away – it is still a trading unit in use nowadays. At least in Portugal eggs come in half a dozen packages.
12 also have another cool property: dividing days in year by days in lunar cycle we get: 365,25 / 28: around 13. 12 is the nearest lower pair number to bridge the sun and lunar cycles. Probably irrelevant…
Ok, this guys gave some thought before choosing their base. But there are other cool number to simplify math. Take the babylonian numerals, based 60. More divisors, cool! And here’s why we 60 seconds in a minute, and 60 minutes in an hour and 360 degrees in a circle.
Finally we use base 10. What to do when we need to multiply by 1⁄3 or 1⁄4? It seems that we chose it simply because we are lazy and prefer counting fingers… :S
And then we have computers, but we all know that, right?
<update>
Here’s a divisor’s list (if I had to quickly bet on successful bases, I’d bet on the bold ones):
1 ( 1) 1
2 ( 2) 1 2
3 ( 2) 1 3
4 ( 3) 1 2 4
5 ( 2) 1 5
6 ( 4) 1 2 3 6
7 ( 2) 1 7
8 ( 4) 1 2 4 8
9 ( 3) 1 3 9
10 ( 4) 1 2 5 10
11 ( 2) 1 11
12 ( 6) 1 2 3 4 6 12
13 ( 2) 1 13
14 ( 4) 1 2 7 14
15 ( 4) 1 3 5 15
16 ( 5) 1 2 4 8 16
17 ( 2) 1 17
18 ( 6) 1 2 3 6 9 18
19 ( 2) 1 19
20 ( 6) 1 2 4 5 10 20
21 ( 4) 1 3 7 21
22 ( 4) 1 2 11 22
23 ( 2) 1 23
24 ( 8) 1 2 3 4 6 8 12 24
25 ( 3) 1 5 25
26 ( 4) 1 2 13 26
27 ( 4) 1 3 9 27
28 ( 6) 1 2 4 7 14 28
29 ( 2) 1 29
30 ( 8) 1 2 3 5 6 10 15 30
31 ( 2) 1 31
32 ( 6) 1 2 4 8 16 32
33 ( 4) 1 3 11 33
34 ( 4) 1 2 17 34
35 ( 4) 1 5 7 35
36 ( 9) 1 2 3 4 6 9 12 18 36
37 ( 2) 1 37
38 ( 4) 1 2 19 38
39 ( 4) 1 3 13 39
40 ( 8) 1 2 4 5 8 10 20 40
41 ( 2) 1 41
42 ( 8) 1 2 3 6 7 14 21 42
43 ( 2) 1 43
44 ( 6) 1 2 4 11 22 44
45 ( 6) 1 3 5 9 15 45
46 ( 4) 1 2 23 46
47 ( 2) 1 47
48 (10) 1 2 3 4 6 8 12 16 24 48
49 ( 3) 1 7 49
50 ( 6) 1 2 5 10 25 50
51 ( 4) 1 3 17 51
52 ( 6) 1 2 4 13 26 52
53 ( 2) 1 53
54 ( 8) 1 2 3 6 9 18 27 54
55 ( 4) 1 5 11 55
56 ( 8) 1 2 4 7 8 14 28 56
57 ( 4) 1 3 19 57
58 ( 4) 1 2 29 58
59 ( 2) 1 59
60 (12) 1 2 3 4 5 6 10 12 15 20 30 60
</update>

.jpg)

 Here’s the next book I have to read:
Here’s the next book I have to read: 

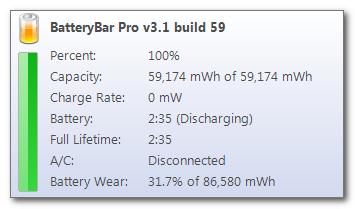
 I’ve been trying to geotag my photos using my PDA so I can cross-reference them by date. Though the process works it wastes by PDA’s battery on something like 1 1/2 hours.
I’ve been trying to geotag my photos using my PDA so I can cross-reference them by date. Though the process works it wastes by PDA’s battery on something like 1 1/2 hours.